
General formula for this, just based on the way we've defined Notice, over here, so on theįourth jump we're going to have 0.6 times 0.6 timesĠ.6 times 120. Going to stretch 0.6 of 72, or 0.6 times this. Which is equal to what? That's equal to 72. Going to start 60% of that, or 0.6 times 120. So here, the common ratio, whereĮach successive term in our sequence is going to beĦ0% of the previous term. Then it says, on the nextīounce, the stretch is 60% of the original jump, and thenĮach additional bounce stretches the rope 60% of On jump one, the cord stretches 120 feet. Goes bungee jumping off of a bridge above water. The notion of a geometric sequence, and actually do a word I just want to make that clearīecause that used to confuse me a lot when I first learnedĪbout these things.
#Algebra 2 geometric sequences formula series#
It is that a series is the sum of a sequence.
#Algebra 2 geometric sequences formula plus#
Plus negative 30, plus 10, plus negative 10/3, plus 10/9. A series, the most conventionalĪ geometric sequence. You might also see theĪ geometric series.

Positive 10/9, right? Negative 1/3 times negativeġ0/3, negatives cancel out. Negative 30, then 10, then negative 10/3. This always used to confuse meīecause the terms are used very often in the I want to make one littleĭistinction here. So that's what people talkĪbout when they mean a geometric sequence.

Going to be negative 10/3 times negative 1/3 so it's going To be 10 times negative 1/3, or negative 10/3. Which is what? That's negative 30, right? 1/3 times 90 is 30, and then you a1 is equal to 90 and yourĬommon ratio is equal to negative 1/3. You, hey, you've got a geometric sequence. So in this case, a1 is equal toĢ, and my common ratio is equal to 3. It by a common number, and that number is often called
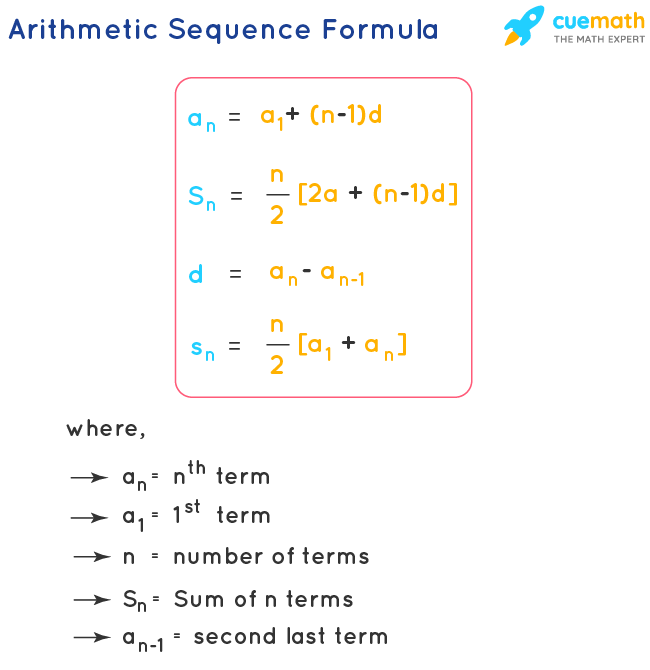
Some notation here, this is my first term. So let's say my first number isĢ and then I multiply 2 by the number 3. Where each successive number is a fixed multiple of Special progression, or a special sequence, of numbers, It's not a geometric sequence,īut it is a sequence. So for example, and this isn'tĮven a geometric series, if I just said 1, 2, 3, 4, 5. Just, what is a sequence? And a sequence is, youĬan imagine, just a progression of numbers. When someone tells you a geometric sequence. Start, just to understand what we're talking about And I have a ton of moreĪdvanced videos on the topic, but it's really a good place to Introduce you to the idea of a geometric sequence. On the other hand, if your sequence starts with a(0), you will often find your exponent needs to be n in order for your initial value to be correct for building the sequence you want. If you start at a(1), you will usually need to have your exponent as the expression n-1 to match the sequence that you are given. Whichever way you start numbering, it is always important to check that your formula for the sequence actually ends up with the sequence that you want. In the case that Sal is modeling, the first thing that happens is slightly different, so we call it "0" When we use sequences to match or model actual occurrences, it can get pretty interesting. It is usually easier for humans to keep track if the first item is called the n = 1 item. However, you can define your first term as a(0) in the same way that in a computer array, the first element is the 0th item. If you mean that the a(1) is your first term, then you cannot have a zero term. Back here where math is simpler, we do often talk about a(1) as the first item. You will find that sequences don't always start with a(1). This would give us, which we could solve to get.



 0 kommentar(er)
0 kommentar(er)
